| Complexity level: | 9 |
| Project cost ($): | 20 |
| Time required: | 1 day to prepare, 1 day for the science project experiment |
| Material availability: | Easily found |
| Safety concerns: | None |
Hypothesis
The frequency of sound from a tuning fork will decrease as the temperature increases.
Overview
Tuning Fork
A tuning fork consists of 2 tines shaped like a ‘U’ and a handle in the middle. When the tines are hit with an object or knocked on the table, they will vibrate. The vibration of the tines will cause the surrounding air to compress and expand creating sound.
The sound generated by the tuning fork is of a specific frequency or tone. This is why the tuning fork is very useful in tuning musical instruments.
The frequency generated by the tuning fork will depend on the material it is made of and also its dimensions.
F = R x √ (E x ρ) / (π x l 2)
F = frequency of the fork, l = length of the tines, E = fork materials Young’s modulus
ρ = density of the material, R= radius of the prongs
Scientific Terms
Materials
The materials required for this science fair project:
- A tuning fork (640 Hz)
- A sound sensor and scope
- A beaker
- Some water
- A stove
- A refrigerator
- An infrared thermometer
Procedure
1. For this experiment, the independent variable is the temperature of the tuning fork. The dependent variable is the resonant frequency of the tuning fork. This is determined by measuring the frequency with a frequency sensor and scope. The constants (control variables) are the resonant frequency of the tuning fork, the material of the tuning fork and the dimensions of the tuning fork.
2. The tuning fork temperature will be varied at 0°C, 25°C, 50°C, 75°C and 100°C.
3. The tuning fork is kept overnight in the freezer of the refrigerator so that its temperature will be 0°C. The next day, the frequency sensor and scope are first set up to take the measurements. The tuning fork is taken out of the freezer and the temperature is checked with the infrared thermometer. After confirming the temperature, a slight knock is made on the tines and the frequency is measured and calculated on the scope. The temperature and frequency of the tuning fork are recorded in the table given below.
4. The tuning fork is allowed to return to room temperature of 25°C and a slight knock is made again on the tines and the frequency is measured and calculated on the scope. The temperature and frequency are again recorded in the table given below.
5. The beaker is filled with water and placed over the stove. The tuning fork is placed inside the beaker. Once the temperature of the water reaches 50°C, the tuning fork is taken out of the water and procedure 4 is repeated.
6. Procedures 4 and 5 are repeated at 75°C and 100°C and the readings are recorded in the table.
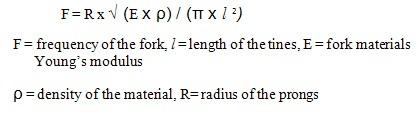
Results
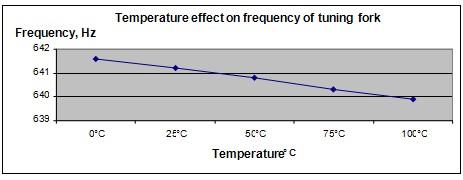
It is observed that when the temperature of the tuning fork is increased, there is a slight reduction in the frequency sound generated.
| Temperature, °C | 0°C | 25°C | 50°C | 75°C | 100°C |
| Frequency, Hz | 641.6 | 641.2 | 640.8 | 640.3 | 639.9 |
Use the graph below to plot the results of the above observations.
Conclusion
The hypothesis that the frequency of sound from a tuning fork will decrease when the temperature increases is proven to be true. As the temperature of the tuning fork increases, the atoms inside the fork will gain kinetic energy and vibrate more. This will cause the fork to expand and become longer. As can be seen from the formula given in the overview section, the frequency of the tuning fork is inversely proportional to the square of the tuning fork length. Therefore any slight increase in the tuning fork length will cause a reduction in the sound frequency produced.
Tuning forks are not only used to calibrate musical instruments anymore. They are also used by doctors to evaluate patients hearing, calibrating speed detection radar and in temperature measurement devices.
Also consider
What would happen if the experiment was repeated by using a tuning fork made from a different material?
The experiment can be repeated using tuning forks of different frequencies.
References
Tuning Fork - http://en.wikipedia.org/wiki/Tuning_fork
Longitudinal waves and tuning forks - http://www.physicsclassroom.com/mmedia/waves/tfl.cfm

